Generally one can say that the closer the spheres, the larger and brighter are the images. This is to be expected although I have not been able to determine a quantitative relationship. Qualitatively one knows that the reflected light that reaches the film plane and makes an image is proportional to the illumination reaching the object (which depends upon the optical power output of the flash multiplied by the "radiation pattern" factor), to the reflectivity of the object, to area of the lens aperture and to the inverse fourth power of the distance (just as with radar - inverse square out t the target and inverse square back to the receiver). The inverse fourth power with distance means that the image brightness (actually the image exposure, which is the product of the optical power per unit area within the image multiplied by the exposure time) changes rapidly with distance of the reflective object. On the other hand, the image size also decreases with increasing distance, almost in the inverse proportional to distance (even though the object is too close for to be focused) so the image area is approximately proportional to the inverse square of the distance. Therefore the combination of the inverse fourth power decrease of illumination on the image with the inverse square shrinkage of the image area means that the exposure (proportional to the power per unit area) decreases only as the inverse square of the distance. Both the overall decrease in image size and brightness with increasing distance is evident in Figure 5. However, distance alone does not explain the brightness variation. The image brightness is also affected by the object size and this means that a collection of different sized objects all at the same distance will make images approximately the same size but differing considerably in brightness. The size dependence of the brightness occurs because the amount of light reflected by one of these tiny objects is proportional to its "cross-sectional area," that is, to its diameter squared. In the case of these glass spheres there was a wide range in diameters and hence a wide range in image brightness even for spheres at nominally the same distance.

(B) FLASH DURATION

The shape of an image of a moving object is determined by the object shape itself as modified by motion during the exposure time. Hence, if a perfectly circular light or steady intensity moved in a straight line a distance 3 times its own diameter during the exposure time the resulting image would be elongated, 4 times as long as it is wide, with rounded ends.(Why not 3 times its own width? Draw a circle on a piece of paper. It has some diameter, d. Now imagine sliding the circle to the right by the distance d, and then another distance d and then once more. Now measure the distance from the far left to the far right boundary. It is d + md, where m is the number of displacements.) In the case of a constantly moving object with a constant velocity v perpendicular to the line of sight the length of the image is d + vt. (In the previous example vt was 3 times the diameter, 3d, so we had d+3d = 4d.) (In the more general case the length of the image is the integral of the component of velocity perpendicular to the sighting line over the time of the exposure.) Clearly the shorter the exposure time the smaller the motion "smear." In order to determine how much of the image shape might be due to motion it is necessary to know the exposure duration. This duration is determined by the shutter during ordinary non-flash photography and by the flash duration when a flash is used.

I set up an experiment to measure the shutter time (time during which the shutter is open), the flash duration and the synchronization between the shutter and flash of a recyclable camera. This experiment used a steady light illuminating the lens and a photodiode to measure the light transmitted. (Note: this experiment required partial destruction of the camera since I wanted to place the light source just behind the lens/aperture/shutter combination.) I operated the shutter several times without the flash and I also operated the shutter with flash. Figure 6 shows the results. The near-trapezoidal shape of the two "shutter graphs" at the left side is expected from the mechanics of the shutter: it opened quickly but not instantaneously, remained fully open for a period of time and then quickly closed. (The downward slope of the "top" of the shutter curve is not a result of the shutter starting to close slowly but rather an artifact - a capacitive effect - of the electronic circuitry.) According to the measurements the shutter was fully open for about 8 ms (1/125 of a second, which is a standard camera shutter time for general use). The illustration at the right side of the figure shows the shutter-time curve with the much shorter flash "spike" superimposed. (This was obtained by letting light from the flash reach the photodiode.) Notice that the flash began about 3.5 milliseconds after the shutter started to open and hence about 1.7 ms after the shutter was completely open.

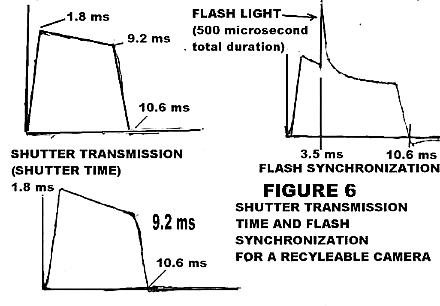
Figure 7 illustrates the time dependence of the flash intensity for the recyclable camera and also for the Polaroid Model 600. The amplitudes of the two flash intensities were about the same, but obviously the Polaroid flash was shorter.

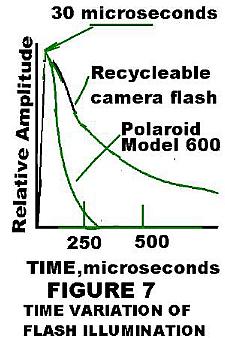
For the recyclable camera the flash intensity reached its peak very, very quickly (about 30 microseconds) and then the flash brightness decayed (approximately exponentially) over the next millisecond. The effective duration of the flash was about 300-500 microseconds (depending upon how one wants to quantitatively define "effective duration"). When photos are taken in the dark the only source of light is the flash and hence the flash duration determines the exposure time. (In normal non-flash daylight shots the shutter determines the exposure time.) This is an "effective shutter time" of 1/2000 to 1/3000 of a second. An object moving several meters per second or millimeters per millisecond will be quite effectively "stopped" in its motion by such a short shutter. By "stopping the motion" is meant having such a short exposure that the image hardly moves during the exposure. For example, if a tiny object were to move perpendicular to the line of sight at 1 meter per second at a distance of 10 cm from the camera lens its angular rate would be (100 cm/sec)/(10 cm) = 10 rad/sec. For a 3 cm focal length this transfers to an image velocity of 30 cm/sec. In 1/3000 of a second the image would move 30 x (1/3000) = 0.01 cm = 0.1 mm. At the same time, these experiments suggest that the image diameter for a tiny object 10 cm from the lens (of the recyclable type of camera) would be a bit over 1 mm (see above). Hence the motion smear would be a small fraction of the image size and the image would be nearly circular. Objects moving more slowly than 1 m sec or objects at greater distance would create even less smear. (However, objects at greater distance also make smaller images so for constant sized objects at the same velocity but at varying distances the percentage of the image which is smear could be constant.) In the case of the glass spheres used in these experiments the velocities were in the range of several to ten centimeters per second rather than a meter per second so the motion smear is not detectable.

(C) ORBTUBES

Besides the individual circular (or other shaped, depending upon the aperture shape) "orb's" discussed above there have also been photographic recordings of "orbtubes" or "light tubes" such as illustrated in Figure 8 (see also the "orbsite" for further examples).

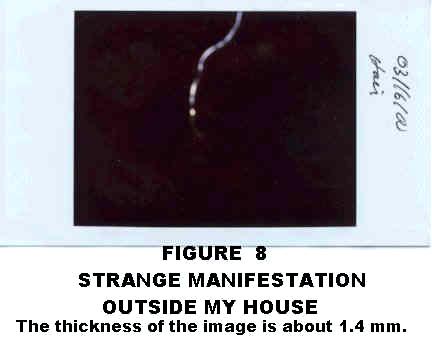
These orbtubes are generally curved, generally have brightness variations along the image and generally shrink in width from one end of the image to the other, such as illustrated in Figure 9 where the image width shrinks from about 4 mm to about 2 mm. The appearance of images such as these naturally led to the speculation that they were evidence of rapid motion of the "orb" or reflector during the time of the flash. However, as the above discussion shows, at speeds typically expected of insects or particulate matter in the air there would not be enough time during the flash for the object to create a long exposure track on the film.

If the length of an orbtube could not be reasonably explained as due to rapid motion of a tiny "point" reflector, then it must be a result of reflection from an elongated reflector. Again the reflector had to be very tiny and close to the lens. To test this hypothesis I purchased a Polaroid "Joycam" which uses type 500 film. The flash is 2.5 inches from the lens and the effective focal length is about 105 mm. The images in photos 8, 9 and 10 are of hairs which were about 4.5/1000 of an inch or about 115 microns (0.115 mm) thick, by measurement with a micrometer. I also sacrificed several of my remaining hairs (sniff) to provide thin elongated objects. Figure 8 shows a hair about a foot from the lens. Figure 9 shows a hair that was held by my fingers while I blew on it to force the free end to be farther from the lens than the near end. The width of the hair image shrinks from about 4 mm to about 2 mm and the brightness is much lower at the far end, except for a small "dot" image just at the end, suggesting that there was a tiny piece of dust that increased the reflectivity at that point on the hair. Figure 10 shows a hair suspended between two milk bottles (!) at 30 cm = 300 mm from the lens. The thickness of the hair image is about 1.9 mm. Other photos taken at shorter distances confirm the approximate relation that the image width is inversely proportional to the distance (3.7 mm at about 150 mm, 5 mm at about 75 mm and 10-12 mm wide at 50 mm). This leads to the following approximate quantitative relation: w = 550 mm^2/D for this 105 mm focal length camera, which can be compared with the previous equation (see above) for the image width using the 31 mm focal length camera, w = 70 mm^2/D.

Figure 10 was taken outdoors at night in a mist. In the photo are lots "orb" images caused by reflections from tiny water droplets. There is also a "haze" or glare over the photo which is probably a result of the very bright reflection from the white plastic milk bottles that supported the hair (which was glued at each end to a bottle).

The hairy experiments show that a very thin, elongated reflector close to the lens can create a wide elongated image of varying brightness and width. There are similarly shaped airborne objects in nature: animal hair, plant seeds borne by thin "hair" and spider web strands, for example. Thus, outdoors one might have plant or animal (spider) generated "hairs" that could blow past the camera, and indoors one could have tiny cloth strands or animal "hairs" that would be temporarily suspended by the atmosphere. Of particular interest are the spider web strands since they can be quite long.

For comparison see an orb tube photograph taken by Bryan Williams (Sargel 18) at.

[Might be a picture of a human hair or spider web strand drifting in front of the camera lens. Note how the lower right portion is more out of focus, being closer to the lens. Also note the similarity to the images taken by Maccabee. It is necessary to rule out a mundane cause such as a hair from the photographer's head being blown by wind in front of the camera before any "intelligence" or paranormal significance can be suspected, let alone attached to such images. That's the scientific method!]

CONCLUSION: ORBS OR DUST